Let B Be A Positive Constant ( B 0 ). Determine Where The Following Functions Are Continuous - Continuity Over An Interval Video Khan Academy / Determine where the function is continuous.

Let B Be A Positive Constant ( B 0 ). Determine Where The Following Functions Are Continuous - Continuity Over An Interval Video Khan Academy / Determine where the function is continuous.. For each of the following determine c so that the function can serve as a pmf of a random. A function is continuous when its graph is a single unbroken curve. Is a continuous function (although to prove it. .positive constant (6 > 0). Velocity, v(t), is a continuous function of time t.

.positive constant (6 > 0). This page is intended to be a part of the real analysis section of math online. Then f ∈ s, but 2f ∈ s. The standard deviation must be 1. So you may take distant points and map them to nearby points.

5 2 Exponential Functions Mathematics Libretexts from math.libretexts.org

F is continuous at x = 0. Introduced above is a continuous function on. How we can understand that function is continuous? You don't need to worry about validation, since arr1 and arr2 must be arrays with 0 or more integers. Velocity, v(t), is a continuous function of time t. All elementary functions are continuous at any point where they are defined. Let $f$ be a function defined by. Continuous as in defined, and because this function is a rational one, it is undefined where ever the denominator is 0.

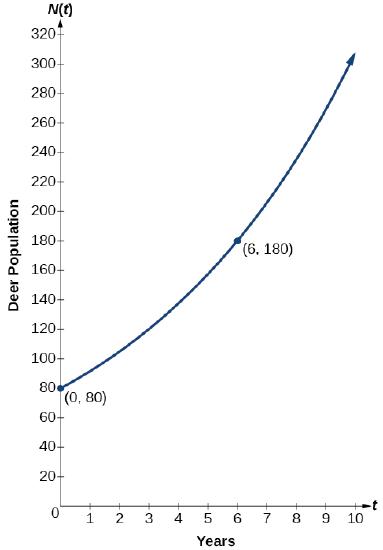
The linear combination of continuous functions.

The last three examples depicted functions there were continuous on all of $\mathbb{r}$. That is not a formal definition, but it helps you understand the idea. An elementary function is a function built from a finite number of compositions and combinations using the four operations (addition, subtraction, multiplication, and division) over basic elementary functions. So you may take distant points and map them to nearby points. Number of point of discontinity point in interval. For a function to be continuous at x = a, lim f(x) as x approaches a must be equal to f(a) and obviously the limit must exist and f(x) must be which of the following is also true? Which of the following is not a characteristic of the normal probability distribution? Although $g$ is not monotone, it can be divided to a finite number of regions in which it is monotone. The following definition means a function is continuous on a closed interval if it is continuous in the continuous functions are where the direct substitution property hold. Using continuity to calculate limits. Which of the following statements about f is false? The graph of a continuous function can be drawn without lifting the pencil from the paper. Take a constant function f (x) = 1 for all x.

The last three examples depicted functions there were continuous on all of $\mathbb{r}$. Discuss the differentiability of `f(x) = arc sin 2x / (1 + x^2)`. The returned value must be a string, and have *** between each of its letters. This is not always the case though. Continuous as in defined, and because this function is a rational one, it is undefined where ever the denominator is 0.

Continuity And Ivt from i.ytimg.com

This page is intended to be a part of the real analysis section of math online. Look out for holes, jumps or vertical asymptotes (where the function heads up/down towards infinity). If a function f (x) is continuous on its domain and if a is in the domain of f , then. Learn how to find the value that makes a function continuous. Where m is a constant. Is assumed to be continuous in general, this function is also continuous for a special case of an lhs indicated by the following relations Here $y=g(x)$, where $g$ is a differentiable function. This fact can often be used to compute the.

The distribution is symmetrical d.

Velocity, v(t), is a continuous function of time t. I) it is a discrete variable that can only assume values x 1 ,x 2., xn. An elementary function is a function built from a finite number of compositions and combinations using the four operations (addition, subtraction, multiplication, and division) over basic elementary functions. That you could draw without lifting your pen from the paper. The last three examples depicted functions there were continuous on all of $\mathbb{r}$. The idea behind most of them is this: If a function f (x) is continuous on its domain and if a is in the domain of f , then. Number of point of discontinity point in interval. T where k is a positive constant. The following definition means a function is continuous on a closed interval if it is continuous in the continuous functions are where the direct substitution property hold. Then f ∈ s, but 2f ∈ s. For each of the following determine c so that the function can serve as a pmf of a random. Rigorously you need calculus not linear algebra).

Number of point of discontinity point in interval. For each of the following determine c so that the function can serve as a pmf of a random. Similar topics can also be found in the calculus section of the site. A function is continuous when its graph is a single unbroken curve. F is differentiable at x = 0.

Solved 2 A Function F Is Left Continuous At A Point X Chegg Com from media.cheggcdn.com

Logarithmic functions, (x) = log, (x). The distribution is symmetrical d. This fact can often be used to compute the. (e) all f that are continuous. A function is continuous when its graph is a single unbroken curve. Take a constant function f (x) = 1 for all x. Let $f$ be a function defined by. An elementary function is a function built from a finite number of compositions and combinations using the four operations (addition, subtraction, multiplication, and division) over basic elementary functions.

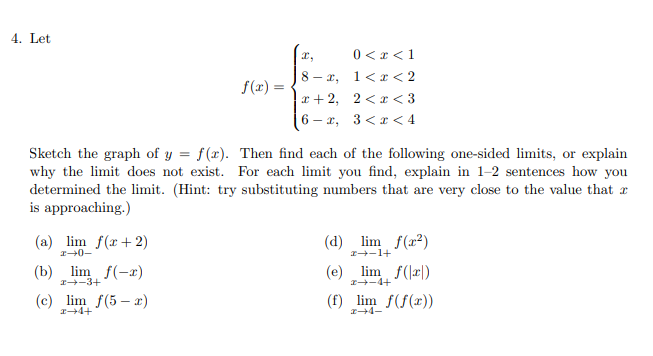
Other functions have points at which a break in the graph occurs, but satisfy this property over intervals contained in we begin our investigation of continuity by exploring what it means for a function to have continuity at a point.

Other functions have points at which a break in the graph occurs, but satisfy this property over intervals contained in we begin our investigation of continuity by exploring what it means for a function to have continuity at a point. Take a constant function f (x) = 1 for all x. This page is intended to be a part of the real analysis section of math online. T where k is a positive constant. A continuous function must take nearby points to nearby points, but it's not under any obligation to do anything with distant points. Which of the following statements about f is false? Similar topics can also be found in the calculus section of the site. Determine where the following functions are continuous: Determine where the function is continuous. Is a continuous function (although to prove it. The idea behind most of them is this: Discuss the differentiability of `f(x) = arc sin 2x / (1 + x^2)`. Learn how to find the value that makes a function continuous.

Source: s3-us-west-2.amazonaws.com

Let $f$ be a function defined by. Velocity, v(t), is a continuous function of time t. Determine where the function is continuous. We provide the following guideline for determining the continuity of a function. Let f be the function given by f(x) = 3e2x i.

Source: media.cheggcdn.com

A continuous function must take nearby points to nearby points, but it's not under any obligation to do anything with distant points. Sal is asked which of the following two functions is continuous at x=3: All elementary functions are continuous at any point where they are defined. How we can understand that function is continuous? T where k is a positive constant.

Source: math.libretexts.org

Here $y=g(x)$, where $g$ is a differentiable function. Let $f$ be a function defined by. Let $x$ be a positive continuous random variable. Look out for holes, jumps or vertical asymptotes (where the function heads up/down towards infinity). 2) let x be a continuous random variable with pdf.

Source: s3-us-west-2.amazonaws.com

Where m is a constant. Determine where the following functions are continuous: A function is continuous when its graph is a single unbroken curve. Since differentiability is a stronger condition than continuity, all differentiable functions are also continuous over the differentiable interval. Discuss the differentiability of `f(x) = arc sin 2x / (1 + x^2)`.

Source: math.libretexts.org

F has an absolute minimum at x = 0 82. The returned value must be a string, and have *** between each of its letters. Logarithmic functions, (x) = log, (x). 2) let x be a continuous random variable with pdf. If a function f (x) is continuous on its domain and if a is in the domain of f , then.

Source: i.ytimg.com

This fact can often be used to compute the. The mean of the distribution can be negative, zero, or positive c. Let's think about the situation where we are approaching three from the right hand side, from values. Questions on the concepts of continuity and continuous functions in calculus are presented along with their answers. Then f ∈ s, but 2f ∈ s.

Source: media.cheggcdn.com

Learn how to find the value that makes a function continuous. The linear combination of continuous functions. The standard deviation must be 1. Introduced above is a continuous function on. That is not a formal definition, but it helps you understand the idea.

Source: s3-us-west-2.amazonaws.com

How we can understand that function is continuous? Similar topics can also be found in the calculus section of the site. For a function to be continuous at x = a, lim f(x) as x approaches a must be equal to f(a) and obviously the limit must exist and f(x) must be which of the following is also true? F is differentiable at x = 0. In general, the common functions are continuous on all the numbers in their domain.

Source: media.cheggcdn.com

Let $f$ be a function defined by. Other functions have points at which a break in the graph occurs, but satisfy this property over intervals contained in we begin our investigation of continuity by exploring what it means for a function to have continuity at a point. (e) all f that are continuous. When given a piecewise function which has a hole at some point or at some interval, we fill. Since differentiability is a stronger condition than continuity, all differentiable functions are also continuous over the differentiable interval.